Use Continuity to Evaluate the Limit Lim X→3 X 13 ˆ’ X2
3) A) Use continuity to evaluate the limit. limx→𝜋. 6 sin(x + sin(x)) B) Use continuity to evaluate the limit. lim x→1 C) Use continuity to evaluate the limit. (Round your answer to three decimal places.) lim x→5 arctan (x2 − 25/5x2 − 25x)
Related Question
Evalutate each limit
Discussion
You must be signed in to discuss.
Video Transcript
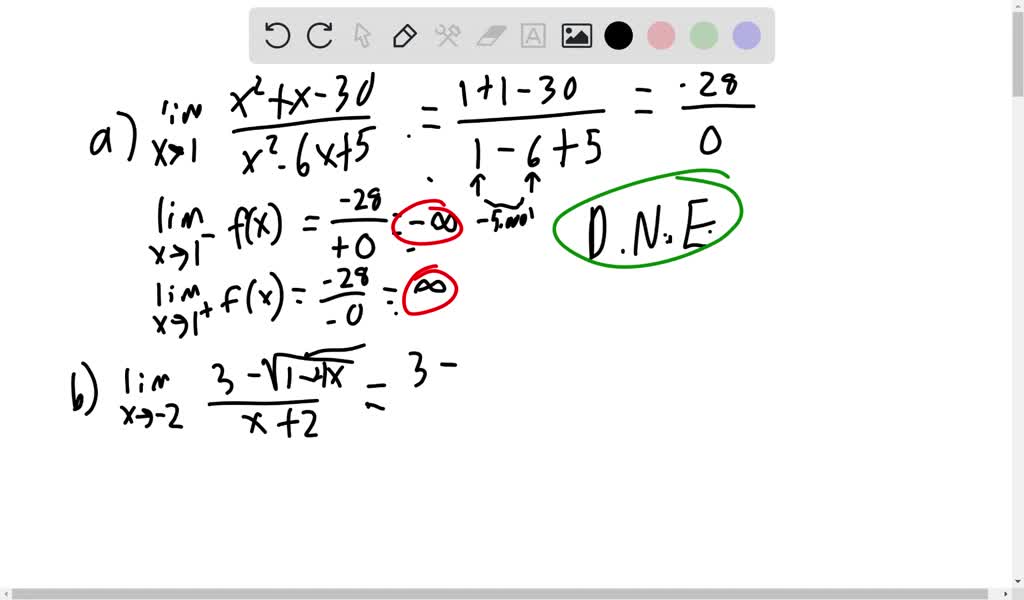
let's take a look at a few limits for part A we're going to say that we have the limit As X approaches one of x squared plus X minus 30 over X squared minus six, X plus five. Now when you evaluate a limit, all you have to do is plug it in. If you get something like zero divided by zero, that means do more work. But if you get a number that's just it. So here if I just plug it in I'm gonna plug in one. So I get one plus one minus 30 Over 1 -6 Plus five. So I get one plus one minus 30 is negative 28 divided by one minus six is negative five plus five is zero. So this means the answer is going to be not a number, it's going to be infinite in some way. However, we have to do a little bit of work to figure out what the actual answer is. The limit as X approaches one from the laugh of my function, I'm just gonna write f of X just because I don't want to write it all again. Well I'm going to plug in a number slightly less than zero. So that means that this number It's just a little bit above one and this one is going to be just a little bit off as well. So from the left we're actually gonna get this number to be a little bit bigger than negative five. So this is more like negative five point something Plus five. So this is actually going to be negative 28 Divided by a positive zero. So this is gonna be negative infinity. Now if we take a look at part B or sorry if we take a look at the limit as X approaches one from the right of F of X. We're going to get basically the opposite will get -28 over a negative zero which is infinity. So overall, since these don't exist, the limit does not exist and that's the answer to part A if we look at part B this one's a little more interesting here we have something we need to rationalize because if I'm gonna plug in negative two, I want to find the limit as X approaches negative too of three minus the square root of one minus four. X All over X-plus two. If I plug in my negative two I get 3 -3 the square root of nine over negative two plus two which is zero divided by zero. This tells me let's do more work. So I'm going to multiply by the conjugate. So I have the limit As X approaches -2 of What happens if I multiply this thing by its conjugate which is 3-plus the square root of 1 -4 x. We'll do the same thing on top and bottom. When I multiply by the conjugate, I'm just gonna square the first part -12 Part like this divided by Well on the bottom it's just these parts I can't really do much with it but that's okay because this gives me the limit as X approaches negative too of 9 -1 is eight plus four. X over X plus two times three plus the square root of one minus four X. Now here eight plus four X. I can pull out a two or sorry four and it's I'm left with X plus two. So really this is just the limit as X approaches -2 of To over three plus the square root of 1 -4 x. and now I can plug the negative two in to get to sorry To over three plus the square root of nine is 3. So that's 2/6 or 1/3. Now let's take a look at part. See for C we have the limit As X approaches -3 of X-plus three Over the 4th root Of X -5 Plus two. Now my first thought here is that fourth root is going to cause us some problems because it's kind of hard to multiply by the conjugate for 1/4 root. So instead I'm going to note that if I take X and plug in negative three, I'm going to get the 4th root of -8. So -3 is nowhere near the domain because this does not exist. This is undefined. So the whole limit does not exist because we're outside of our domain
Source: https://www.numerade.com/ask/question/3-a-use-continuity-to-evaluate-the-limit-limx-6-sinx-sinx-b-use-continuity-to-evaluate-the-limit-lim-x1-c-use-continuity-to-evaluate-the-limit-round-your-answer-to-three-decimal-places-lim-x-11287/
Post a Comment for "Use Continuity to Evaluate the Limit Lim X→3 X 13 ˆ’ X2"